“Fluid volume that would be required to contain the amount of drug present in the body at the same concentration as in the plasma”
Description
According to its definition the volume of distribution (abbreviated Vd) is not a physical space, but a dilution space, or in other words an apparent volume. Nevertheless, the volume of distribution of a drug gives information on its distribution in the body.
The Vd is calculated as the ratio of the dose present in the body and its plasma concentration (see below, “assessment”), when the distribution of the drug between the tissues and the plasma is at equilibrium.
Accordingly, a drug that accumulates in tissues as e.g. fat tissue, will have a relatively low plasma concentration with regard to the administered dose, and consequently, the calculated Vd will be high.
Typical liquid volumes are in % (in L for a man of 70 kg):
| total water: | 60% (50-80%) | 42 L |
| intracellular volume: | 40% | 28L |
| extracellular volume: | 20% | 14L |
| plasma volume: | 4% | 3L |
| blood volume: | 8% | 5.5L |
Chart Pharmacokinetics
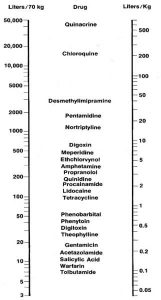
Drugs with a very small Vd (<10 L) are mainly confined to the intravascular fluid, thus the blood, corresponding to roughly twice the plasma volume. This may occur for two reason :
- The molecule is too large to leave this compartment.
- The molecule binds preferably to plasma proteins (e.g. to albumin) and much less to tissue proteins. Competition for plasma protein binding sites can occur between such drugs or with endogenous substances.
Some drugs cannot enter cells because of their low lipid solubility. These drugs are distributed throughout the body water in the extracellular compartment and have a relatively small Vd (12-20 L).
Drugs that accumulate in organs either by active transport or by specific binding to tissue molecules have a high volume of distribution, which can exceed several times the anatomical body volume. Therefore, Vd should not be identified too closely with a particular anatomical compartment. Lipid-soluble drugs are stored in fat. Bone is a reservoir for drugs such as tetracycline and heavy metals.
The figure below shows values of Vd for different drugs (click on the image to visualise an enlarged version):
Clinical implications
The volume of distribution is useful in estimating the dose required to achieve a given plasma concentration as A = C ·Vd, with A = amount of drug in the body ( ≈ dose, shortly after administration) and C = plasma concentration.
Variation of Vd mainly affects the peak plasma concentration of the drug. This is important when peak plasma concentration is essential for the therapeutic effect (e.g. hypnotics). Drug dosage must be adapted to the Vd for such drugs.
Vd varies with individual height and weight. The most important causes of variation of Vd are accumulation of fat (for lipid-soluble drugs) such as for obese patients, or accumulation of fluids (for water-soluble drugs) such as ascites, edema or pleural effusion. As the proportion of each body compartment varies with age, so does the Vd for most drugs.
Assessment
$$Vd = {A \over C}$$
A = amount of drug in the body
C = plasma concentration
Most importantly, the Vd is determined in conditions under which the drug distribution between the plasma and the tissues is at equilibrium.
The Vd is commonly determined as shown below, after injection as an iv bolus. As can be appreciated from the description of the distribution and elimination phases (see corresponding figure), the distribution of the drug between the plasma and the tissues is at an equilibrium, when the elimination phase is reached.
The extrapolated plasma concentration at time 0, C(0), is back-extrapolated from the slope of the elimination phase of the semilogarithmic plasma concentration vs. time decay curve (see figure in Distribution and elimination phases ). C(0) corresponds to the plasma concentration at time 0, if the distribution of the injected drug had been immediate. The amount of drug in the body at time 0 equals the dose administered. Therefore the following equation can be applied:
$$Vd = {D \over C(0)}$$
D = dose
C(0) = extrapolated plasma concentration at time 0